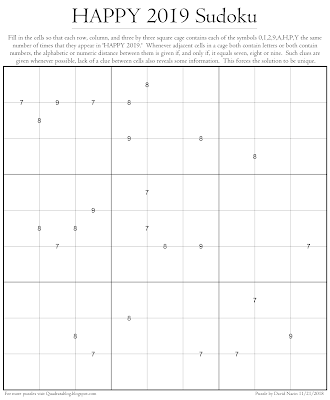
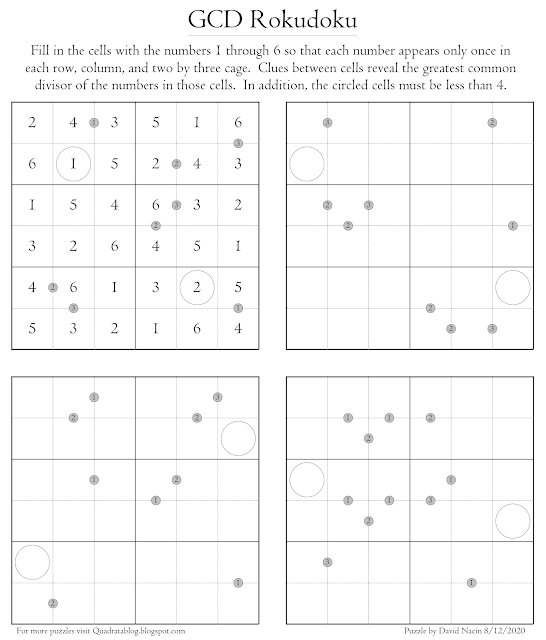
A Rotation Puzzle
For a change of pace from Latin squares, I decided to post a rotation puzzle this week. In each set of objects, there are exactly two of them which are the same, in the sense that you can rotate one to look like the other. The goal is to find the two, and circle both. These puzzles kind of remind me of the ones in children's magazines, where you need to circle what is different in two different drawings. Here instead, you circle what is the same, up to rotation at least.
Link to higher quality PDF.
I've made these over all the platonic solids, but I decided to put out an icosahedra puzzle here first. By making each have four blue and eight yellow corners, it makes it a bit more challenging to distinguish them. It turns out that any group of thirteen such icosahedra must have some the same, as there are only twelve ways to color them in yellow and blue with these numbers of each. These puzzles only have one solution, so you're establishing a list of all the possibilities by solving them.
Link to higher quality PDF.
I've made these over all the platonic solids, but I decided to put out an icosahedra puzzle here first. By making each have four blue and eight yellow corners, it makes it a bit more challenging to distinguish them. It turns out that any group of thirteen such icosahedra must have some the same, as there are only twelve ways to color them in yellow and blue with these numbers of each. These puzzles only have one solution, so you're establishing a list of all the possibilities by solving them.

Comments
Post a Comment